Are Form 8815 Index 2023-2024

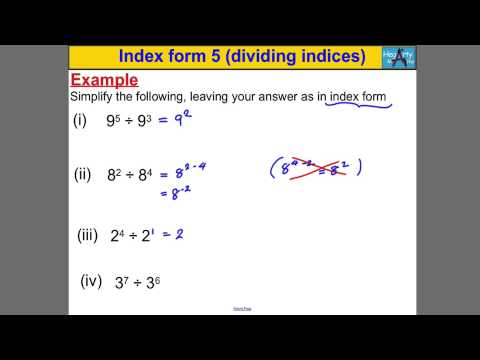
Hi there and welcome to another video from Hegarty Maths. It's Mr. Haggerty here and in this video, we're talking about index form. This is our fifth video where we discuss dividing indices. Before we get started, I want to use a skill that we know from fractions. If someone asked you to evaluate 12/20, what might you do? Well, let me tell you what I would do. I would write it as 12 over 20. I would write the division as a fraction. Then, I would try to simplify this fraction by finding the highest common factor of 12 and 20, which is 4. I would then divide the top and bottom by 4. But do you remember when I first introduced this idea? I said to write it as 4 multiplied by 3 over 4 multiplied by 5. Dividing by 4 is the same as canceling the 4 from the top and bottom, leaving you with 3/5. So, there's a skill I want you to remind yourself of from what we've previously done on fractions. This skill involves canceling a factor on the top and bottom of a fraction or thinking of it as dividing the top and bottom by that factor. Now, let's move on to dividing indices. Suppose someone said, "What is 7 to the power of 5 divided by 7 cubed?" In the same way I just showed you, would you mind if I wrote that as 7 to the 5 over 7 cubed? I hope not. If we actually write out 7 to the 5 as a product and 7 cubed as a product, we would have 7 multiplied by 7 multiplied by 7 multiplied by 7 multiplied by 7 divided by 7 multiplied by 7 multiplied by 7. Now, just like when we were...