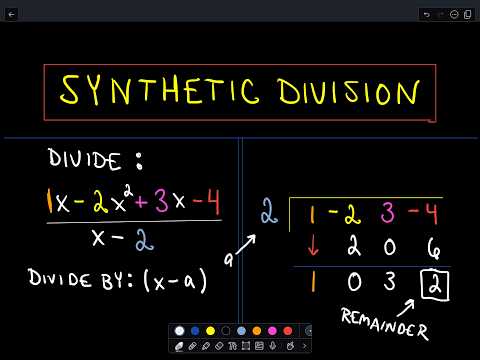
Okay, in this video, I want to do an example of what's called synthetic division. Synthetic division is when you basically divide one polynomial by a first-degree polynomial, so X to the first. It may or may not have a number in there. If it's just X, you would just break things up individually, and it would be pretty easy anyway. Suppose we want to divide X cubed minus 2x squared plus 3x minus 4 by X minus 2. What we do in this case is, whatever we're dividing by, so notice there's a minus 2 (X minus 2), we actually take the opposite sign and we'll use positive 2. That's what we stick outside. Notice I have 1x cubed minus 2x squared plus 3x minus 4. Notice there are no two terms missing. It starts with the third degree, second degree, first degree, and then we just have a number. All we do is write the coefficients. So, one. There's a negative 2 in front of the x squared. There's a positive 3 in front of the X, and there's a negative 4 just hanging out. With synthetic division, what you do is, the first term, you just drop it right down. There's nothing to do. The idea is we multiply. I'm going to take positive 2 times 1 and then stick that number in the next spot. So, positive 2 times 1 will give us positive 2. What we do next is add those numbers together in this column. Negative 2 plus 2 is going to give me 0. We repeat this process. Now, we take positive 2, multiply it by 0, and that's what we stick in the next column. So, positive 2 times 0 will give us 0. If we add those together, we'll get 3....
Award-winning PDF software





Video instructions and help with filling out and completing Fill Form 8815 Div