When Form 8815 Limitations 2023-2024

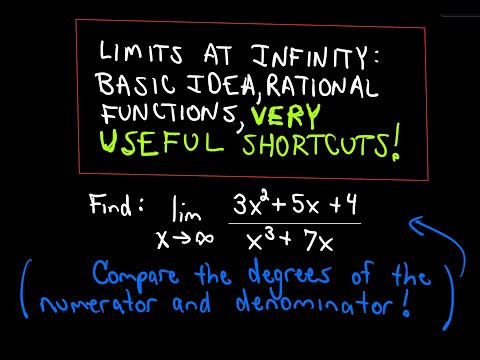
Okay, and in this example (or these examples), we're going to talk about limits at infinity, either positive infinity or negative infinity. The idea is the same as in a regular limit. In this case, with the notation, it means that you're putting in values of x that get larger and larger. So, for example, if I put in 100 into this formula, I'll get a number out. If I put in a thousand, I'll get a number out. If I put in a million, I'll get a number out. And you just keep going and going. The idea is that if the numbers you're getting out are getting closer and closer to something, that's what we say the limit is. In these problems, limits at infinity, I like a little bit better because they're a little more straightforward. The basic ones are a bit more mechanical. Just like anything in math, there are definitely variations on this theme. But when you have a rational function (a polynomial over a polynomial), you always look at the highest power of x in the denominator. So, in this case, my highest power of x is x cubed. What we're going to do is divide every single term in the problem by x cubed. So, I'll get the limit as x goes to infinity. I'll take 3x squared divided by x cubed. I'm going to take 5x divided by x cubed. I'll take 4 divided by x cubed. I'll have x cubed over x cubed. And then, I'll have 7x over x cubed. The next thing we do is simply simplify this down a little bit. So, 3x squared over x cubed, I'll have 1/x left over in the denominator. 5x over x cubed, I'll get 5/x squared. Well, there's not much to do with the...